Fragment molecular orbital calculations
using the RI-MP2 method
(upload: 2010.06.30)
In this page, we give a discription about RI-MP2 method in the FMO method. The following PPTs were used for the previous presentations. This work has been pablished [1].
01
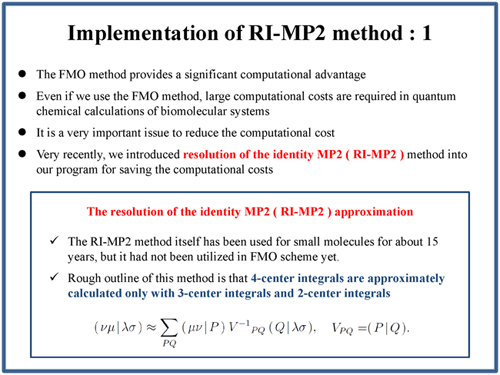
The fragment molecular orbital (FMO) method [2] has a significant computational advantage. However, even if we employ the FMO method, large computational costs are required in quantum chemical calculations of biomolecular systems. Thus, it is a very important issue to reduce the computational cost of the FMO method. Recently, we implemented the resolution of the identity (RI) approximation into our program, PAICS, for saving the computational cost of MP2 calculations in the FMO method. The RI approximation itself has been used for small molecules for about 15 years [3], but it has not been utilized for the FMO method yet. Rough outline of this method is that 4-center integrals are approximately calculated only with 3-center integrals and 2-center integrals, and by which computational const can be reduced.
02
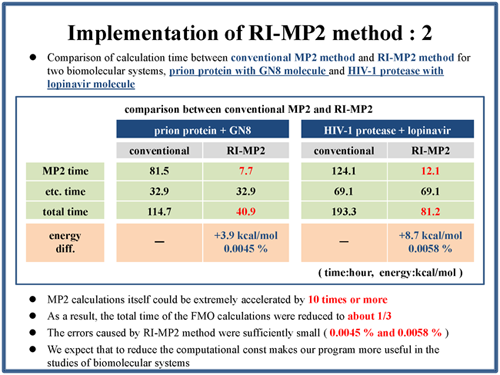
I show the comparison of calculation time between conventional MP2 method and RI-MP2 method for two real biomolecular systems, i.e., the complex of the prion protein with GN8 molecule and the complex of the HIV-1 protease with lopinavir molecule. MP2 calculations itself could be extremely accelerated more than 10 times. For example, in the case of the prion protein, time of conventional MP2 calculation was 81 hours, on the other hand, using RI-MP2 method, it was reduced to about 8 hours. As a result, the total time of the FMO calculations were reduced to about one third. In the case of prion protein, total time was reduced from 114 hours to 40 hours. The errors caused by RI-MP2 method were sufficiently small. In the case of prion protein, the error of the total energy is only 0.0045%. We expect that to reduce the computational const makes our program more useful in the studies of biomolecular systems.
refrence
[1] Fragment molecular orbital calculation using the RI-MP2 method, T. Ishikawa and K. Kuwata,Chem. Phys. Lett., 474 (2009) 195-198 (DOI: 10.1016/j.cplett.2009.04.045)
[2] Fragment molecular orbital method: an approximate computational method for large molecules, K. Kitaura, E. Ikeo, T. Asada, T. Nakano, and M. Uebayasi, Chem. Phys. Lett., 313 (1999) 701-706 (DOI: 10.1016/S0009-2614(99)00874-X)
[3] Use of approximate integrals in ab initio theory. An application in MP2 energy calculations. M. Feyereisen and G. Fitzgerald, Chem. Phys. Lett., 208 (1993) 359-363 (DOI: 10.1016/0009-2614(93)87156-W)